SPAIN'S RECENT FAKE GLOBE FOOTAGE

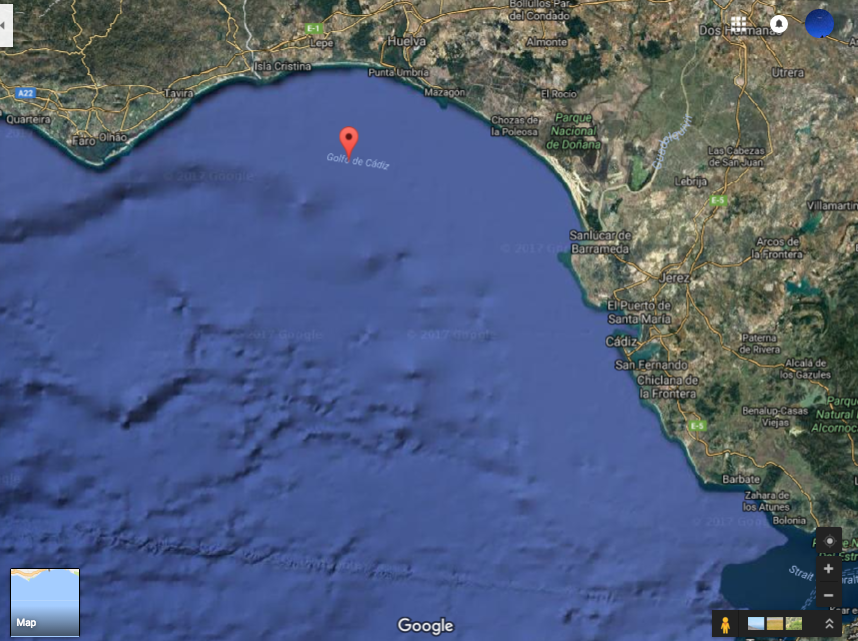
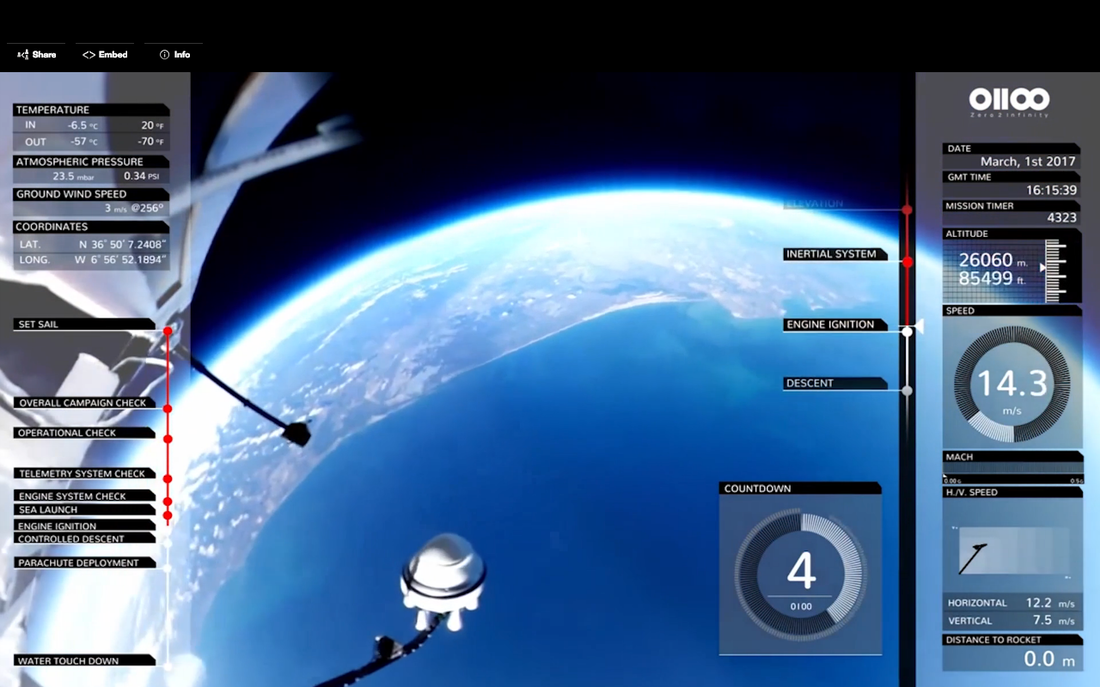
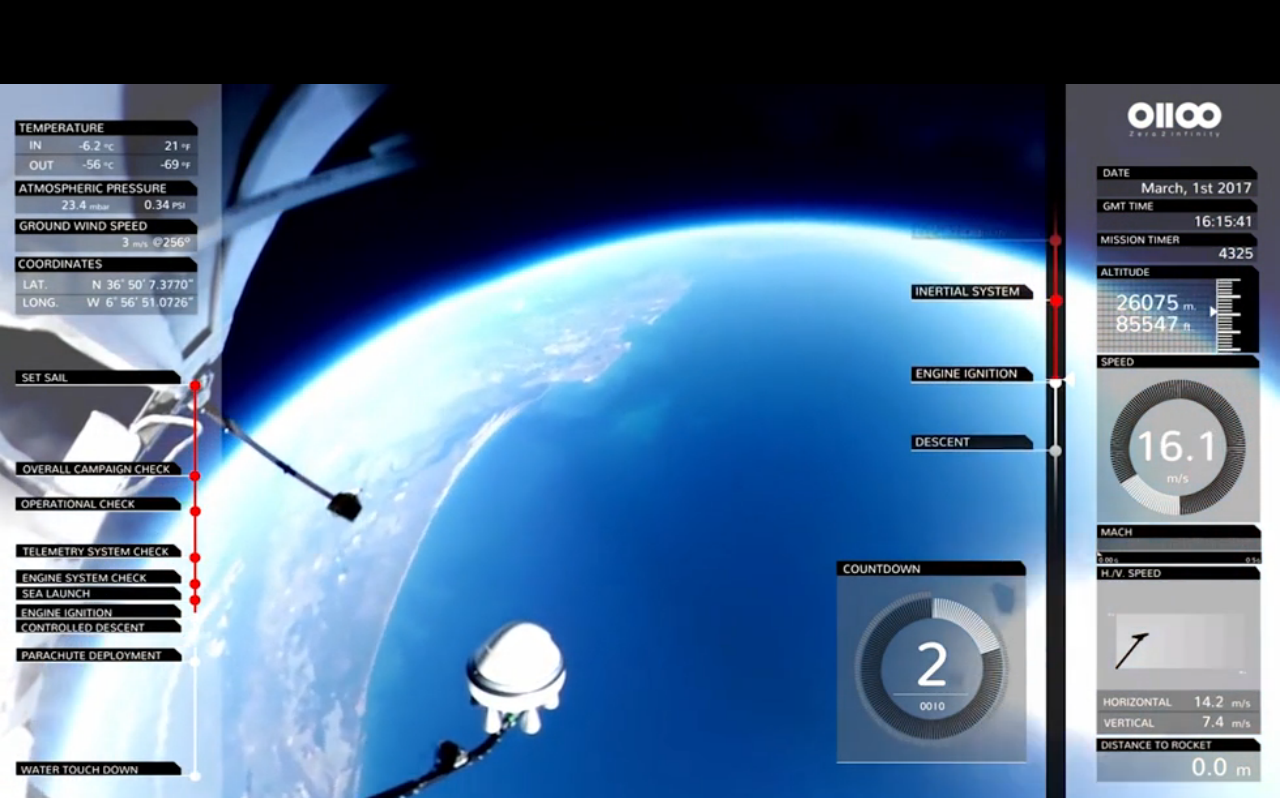
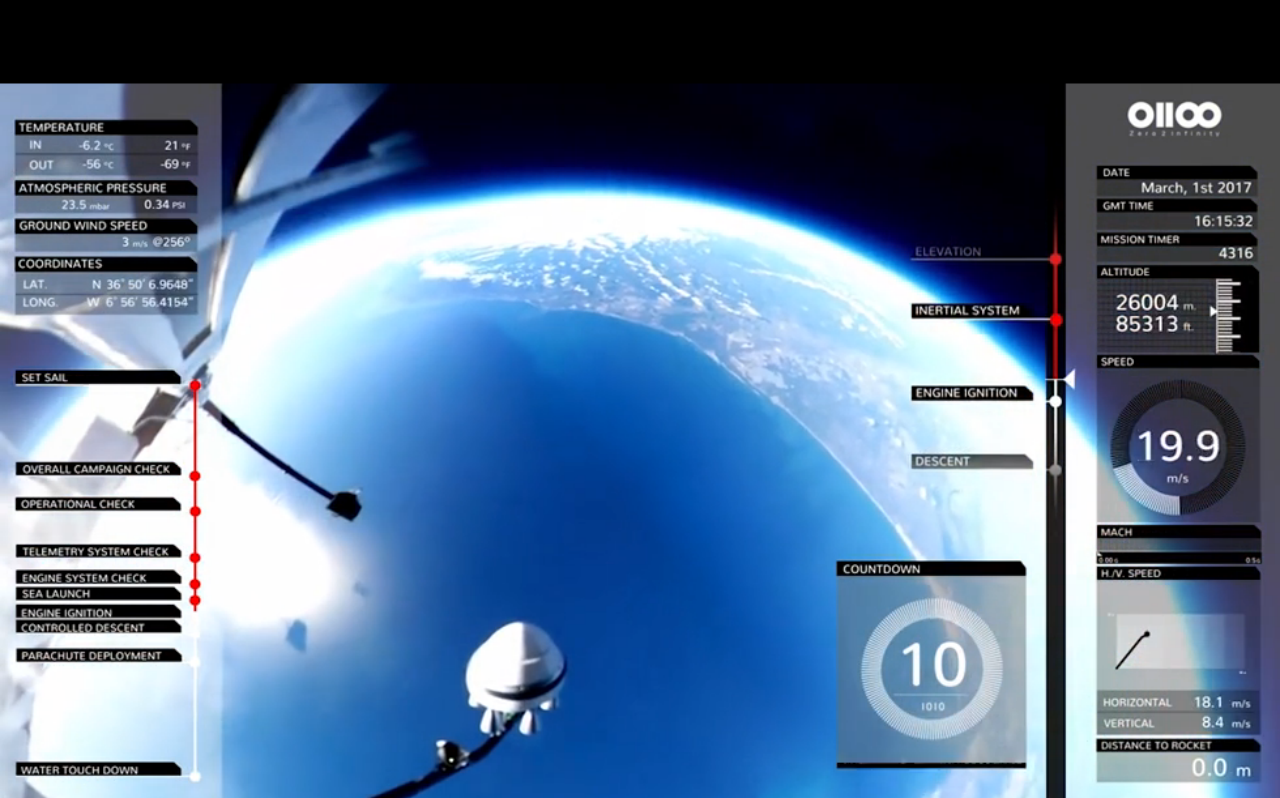
SPACE COMPANY'S FOOTAGE OF GLOBE RAISES QUESTIONS OF SCALE

[SPOILER ALERT: This entire article can be explained in one sentence: watch four seconds of Zero To Infinity's satellite launch video cued up here, and note that your view of the earth is from 15.5 Miles up (25 Kilometers), which is not high enough to see both sides of the globe.]  

On March 1st, 2017, Zero To Infinity, a private Spanish company which "provides access to near space" according to Wikipedia, recently used a hot air balloon to deploy four mini-satellites into orbit. However, the video footage taken from the Bloostar (the hot air balloon) left some unanswered questions with respect to scale....​

Apparently, from a little over 15 miles above sea level you can also see over 10,000 miles of circumferential arc of the globular earth..

Image A. Golf of Cadiz Source: Google Sattelite

Image A (above) is a satellite picture from Google Maps of the Golf of Cadiz, while Image B (below), is from the Bloostar Balloon, from 15.5 miles up. Note the patch of beach on both photos demarcating the same location.

Image B. Gulf of Cadiz. Source: Zero To Infinity

Here's another (Image C) from the footage with the beach patch in better view. 

Image C - Gulf of Cadiz. Source: ZerotoInfinity

To give you a sense of scale, Image D is an As The Crow Flies measurement of the distance between Faro, Portugal, and Cadiz, Spain, which constitutes the majority of the diameter of the Gulf of Cadiz, it comes in at a distance a little under 100 miles (97 m).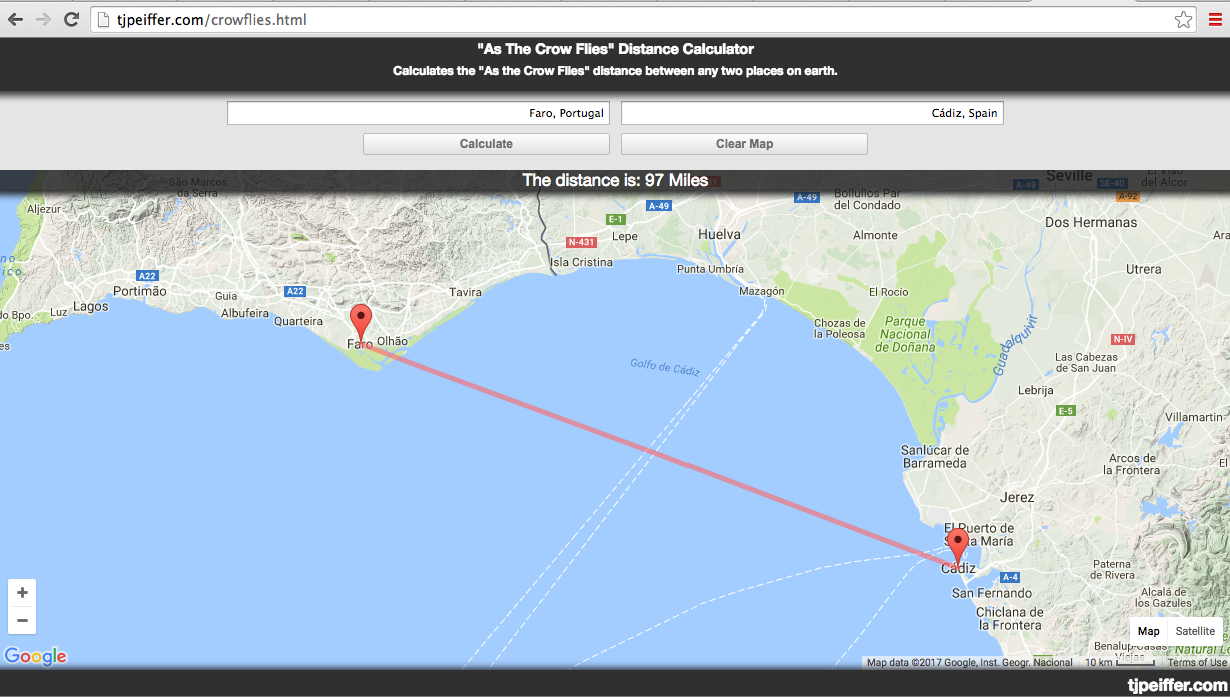 

Image D - Distance from Farro to Cadiz. Source: http://tjpeiffer.com/crowflies.html

---

Here's where it get's interesting. If you imagine that Image B or Image C is a clock face, and it's periphery the outer edge of the clock, note how you can see from about 10 O'clock to 3 O'clock, and 9 O'clock to 2 O'clock, on Images B and C, respectively. This is about 150 Degrees of a 360 Degree Face, or 10,374 Miles of a circle with a give or take circumference of ~24,898 Miles.

Seeing from ten to three is tantamount to observing 10,374 Miles of arc length.

The periphery of Image B depicts 10,374 Miles, while the foreground and central part of the image is consumed by less than 100 Miles. Optically speaking, do you see how egregious it is to assume the exterior of that image can represent 100 times the distance than that of the Gulf of Cadiz in the center of it?

​​Specifically, how can Image B show an arc distance of 10,374 Miles while the distance from Faro to Cadiz constitutes a distance of less than a 100 miles?

What's more, if we were at an altitude which would permit us to see that much earth, Image B is oriented in a North-Westernly direction, and considering the presentation of the arc covers from ten to three on the clock face, The North Pole should be in frame around One O'clock. 

Image E - 10 to 3 on a Clock Face

Not only does its exterior cover over 10,000 miles, the distance between the Golf of Cadiz and The North Pole is 3,668.5 Miles (5,904 Km), or 3,186 Nautical Miles. [Golf of Cadiz is located at 36.9 Degrees North Latitude, and 7.1 Degrees West Longitude, while the North Pole, (theoretically visible in Image B), is located at 90 Degrees North Latitude and 0 Degrees West Longitude.] 

Image F below is another picture from the video footage of the camera yet pointed in the opposite direction as Image B. Here we see a theoretical "bottom to the globe" (Also seen in Image C), around One O'Clock. 

The reason we can presume we're viewing either pole is because "we're far enough away" to be seeing 150 Degrees of circumference of the earth.

The distance from the Gulf of Cadiz to "the South Pole" is a whopping 8,699 Miles (14,001 Kilometers). 

Punch in Lat (36 N, 7 W) and Long (90 S, 0 W) here to see the calculation for yourself.

Image F - "The bottom of the globe" from 15 miles above the Golf of Cadiz.

 Now you may say, yeah but they're way up in Space or something, right?

Well they are admittedly 25 Kilometers (15.5 Miles) up. With respect to how much of the earth's surface they can see in a direction on a spherical earth with a radius of 3,959 miles, it is a simple math equation: [(2rh + h squared) = D squared]

• h=height of line of sight

• r = 3959 miles (radius of the earth)
• d = distance you can see ​

The classic example for this equation is that a person standing at the sea shore, with a line of sight of six feet can see 2.9 Miles before their view collapses into a single point where a ship would "disappear over the horizon."  

Plugging in 15.5 Miles for h, you'll find that distance you can see from a balloon 15.5 miles in one direction is 351.058 miles. In two directions would be twice that or 792.116 miles.

Thus by basic geometry, we can show that from 15.5. miles up at the Golf of Cadiz, beyond 351 miles in any direction, or 792.116 total, one's view of things beyond that at sea level must be blocked by the bulge of the spherical earth with a circumference of about 25,000 Miles. In other words, at that distance, from that altitude the earth should occlude itself, optically speaking, after 351 miles in a given direction. And that occlusion means you would be no where near high enough to see 150 degrees of its total globe shaped circumference. In fact the seven hundred miles you could get from that altitude with your head on a swivel would only amount to viewing 10 degrees of the earth's circumference. 

What that means is that the horizon from 15 miles up, should encompass no more than 10 degrees of circumference, or 700 Miles, of the earth's periphery. 

But in this footage of the purported globe we are seeing over 10,000 miles of circumferential arc, not to mention 3,000 miles to the north pole, over 8,000 miles to antarctica, and all while the diameter of the Gulf of Cadiz, at a measly 100 Miles, fills more than half of the frame!
​
The way the globe is presented, we can presumably see the top of it around 1 o'clock in Image B, and its bottom at the top right of the arc in Images C, and F, however this kind of scope would require a significantly greater altitude than 15.5 Miles.

Note: The Gulf of Cadiz is 3,600 miles from the North Pole, and 8,699 Miles from Antarctica. 

We know the distance from Faro Portugal to Cadiz Spain to be accurately represented at 97 Miles. Considering the scale of this map, does the distance from that patch of sand to the top of the marble look like 3,600 Miles (Image B)? Or does the same spot to the bottom of the marble look like 8,699 Miles (Image F) ? Moreover, wouldn't we need to be hundreds of miles up to gather a scope of the sphericity of the earth, particularly with respect to having viewed both it's northernly and southernly pronounced curves?

According to the current earth shaped model, at an elevation of 15.5 Miles, even with a good camera, the curvature of the earth should begin occlusion at 351 miles.

We're looking at about a 100 Miles in the middle of the frame and over 10,000 on its periphery.

Images Images B, C and F cannot be to scale given the current earth measurements.

May it not instead be footage of what was about 351 Miles of earth, just as the altitude should provide, but where about half of it is the real frame and the other half has been graphically converted into a curved globe and absently dark space? Which begs the question...

Again, note how Image B shows arc from 10 to 3 on a clock, which is 150 Degrees of Circumference, or 10,374 Miles.

Why would someone so deliberately misrepresent the shape of the earth?

Image G - A more plausible degree of earth curvature from 15 miles up shown as the intro to the BlooStar Video Footage.

If the curve in question had only stayed true to how it looks above in Image G, which is far more plausible and looks like 10 degrees, instead of shifting into a blatantly falsified and computer graphically constructed sphere, it probably would have just slipped on by.